The history of the sundial

It is difficult to make assumptions about the age of the sundial. We know, however, that they were already highly regarded 4000 years ago by the Egyptians and Babylonians. Throughout the centuries, they have done their job.

Then, as the wheel clock was invented, it was attempted to manufacture the clock in such a way that it would keep time as well as the supposedly exact running sundial. This didn't' happen because no one believed the sun could make a mistake. The notion of uniform motion was unshakable like a dogma. Motion was only considered to take place in a circle, the ideal curvature. It was, however, long known that the planets traverse their orbits in loop form, which wasn't so easy to explain. It must be said, to honor the ancient astronomers, that they founded an apparent explanation through the overlapping of circular movements by cycloids. They had developed this system to the point that the movements of the heavenly bodies and eclipses could be calculated in advance somewhat precisely.

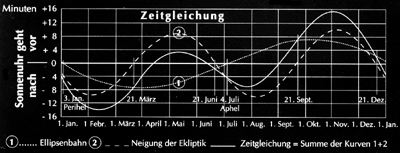
The more precisely the calculation was to be adapted to the actual movement, however, the more complicated the system became, the more circles had to be incorporated and all the more improbable became the whole explanation. Even Kepler tried this out back then. The revolutionary aspect of his idea was letting go of the circular motion dogma and recognizing that the movement of the heavenly bodies was based on elliptical orbits. The elliptical orbit of the earth around the sun is also that which makes it hard on all sundial manufacturers. The varying distance to the sun also results in a varying velocity. But even if the earth traveled at a uniform velocity, the inclination of the ecliptics would distort this motion. The so-called equation of time is calculated from the sum of both errors. It establishes how many minutes every normal sundial runs ahead or behind on a particular day. This value grows to up to a quarter of an hour. If anyone, therefore, wants to know the exact time from a sundial, the value of the time equation valid for this day must first be added to or subtracted from the value read.

The time equation can also be represented as a horizontal loop, a so-called lemniscate. It is often found on dial faces of equatorial sundials.

One may be inclined to say that a sundial is only made to display the sun's true time. To obtain the normal time from this, one should recalculate with the help of the longitudinal difference and time equation. It must, however, be appealing to every sundial manufacturer, to discover a sundial form that carries out this recalculation by itself to compensate for all differences.

The greatest difficulty about this probably is: Every point in the sky at which the sun stands between summer solstice and winter solstice will be reached again between winter solstice and summer solstice. This happens at a different time of day, however. In the case that the sundial should run correctly from summer solstice to winter solstice, it would then have to run incorrectly from winter solstice to summer solstice. The sundial can surely not decide for itself if it is spring or fall.

Several experiments have been made to eliminate this defect, that is, to construct a sundial that compensates for the time equation itself. This leads to a rather complicated, confusing dial face, if the skimpy rod is retained as shadowcaster. Either this, or it becomes necessary to set the value of the time equation that applies to the day before making the reading.

There are sundials that have the dial face set up in the form of a cylindrical jacket around the shadowcaster. At the beginnings of spring and fall, however, the one end of the dial face casts its shadow on the opposite side so that it becomes impossible to read the dial mornings and evenings during these times. Even a sundial on a vertical wall cannot be read the whole day, because even with an ideal southerly position, the wall is shined on in the summer much longer that 12 hours.

Another form is the equatorial sundial where the dial face is mounted at the equator. In the fall and in the winter, however, this dial face is only shined upon on the lower side and during the equinoxes no shadow at all is produced.

You see many different forms, but non of them is without defect. I have now exerted myself to build a sundial that does not have this defect. The following 3 conditions should be fulfilled:

1. Easy reading
2. No shadow of its own, i.e., readable at every moment with unobstructed sunshine
3. Exact time, considering all factors that influence the reading

An international sundial contest furnished the occasion to demonstrate that these conditions were fulfilled, at which time the precision sundial was published by the American astronomical scientific magazine "Sky and Telescope" as one of the three best solutions. The compensation of the time equation is so exact that it makes sense to place minute lines on the dial, due to the fact that, with this form of sundial, it is possible to clearly read the time to the minute.